9.5: Trends in Lattice Energy: Ion Size and Charge
An ionic compound is stable because of the electrostatic attraction between its positive and negative ions. The lattice energy of a compound is a measure of the strength of this attraction. The lattice energy (ΔHlattice) of an ionic compound is defined as the energy required to separate one mole of the solid into its component gaseous ions. For the ionic solid sodium chloride, the lattice energy is the enthalpy change of the process:
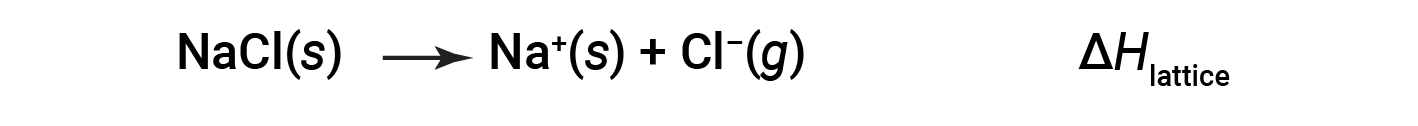
Conventions
Here, the convention is used where the ionic solid is separated into ions, meaning the lattice energies will be endothermic (positive values). Another way is to use an equivalent but opposite convention, wherein the lattice energy is exothermic (negative values) and described as the energy released when ions combine to form a lattice. Thus, make sure to confirm which definition is used when looking up lattice energies in another reference. In both cases, a larger magnitude for lattice energy indicates a more stable ionic compound. For sodium chloride, ΔHlattice = 769 kJ. Thus, it requires 769 kJ to separate one mole of solid NaCl into gaseous Na+ and Cl– ions. When one mole each of gaseous Na+ and Cl– ions form solid NaCl, 769 kJ of heat is released.
Coulomb’s Law and Lattice Energy
The lattice energy ΔHlattice of an ionic crystal can be expressed by the following equation (derived from Coulomb’s law, governing the forces between electric charges):
ΔHlattice = C(Z +)(Z−)/Ro
in which C is a constant that depends on the type of crystal structure; Z+ and Z– are the charges on the ions, and Ro is the interionic distance (the sum of the radii of the positive and negative ions). Thus, the lattice energy of an ionic crystal increases rapidly as the charges of the ions increase and the sizes of the ions decrease. When all other parameters are kept constant, doubling the charge of both the cation and anion quadruples the lattice energy.
Examples
- The lattice energy of LiF (Z+ and Z– = 1) is 1023 kJ/mol, whereas that of MgO (Z+ and Z– = 2) is 3900 kJ/mol (Ro is nearly the same — about 200 pm for both compounds).
- Different interatomic distances produce different lattice energies. For example, compare the lattice energy of MgF2 (2957 kJ/mol) to that of MgI2 (2327 kJ/mol) to observe the effect on lattice energy of the smaller ionic size of F– as compared to I–.
- The precious gem ruby is aluminum oxide, Al2O3, containing traces of Cr3+. The compound Al2Se3 is used in the fabrication of some semiconductor devices. In these two ionic compounds, the charges Z+ and Z– are the same, so the difference in lattice energy depends upon Ro. Since the O2– ion is smaller than the Se2– ion, the Al2O3 has a shorter interionic distance than Al2Se3 and has, therefore, larger lattice energy.
- Another example is zinc oxide, ZnO, compared to NaCl. ZnO has a larger lattice energy because the Z values of both the cation and the anion in ZnO are greater, and the interionic distance of ZnO is smaller than that of NaCl.
This text is adapted from Openstax, Chemistry 2e, Section 7.5: Strengths of Ionic and Covalent Bonds.


