32.8:
RLC Series Circuit: Problem-Solving
Consider an RLC series circuit with a 16 ohm resistor, 0.08 henry inductor, and 0.04 farad capacitor connected to a 120 volt source with a 50 hertz frequency. Determine the circuit's impedance, phase angle, current amplitude, and voltage amplitude across each circuit element.
To solve the problem, identify the known and unknown quantities.
By recalling the reactance equations and substituting the terms, the inductive and capacitive reactance in the circuit can be determined.
Using the impedance equation and substituting the resistance and reactance values, the impedance of the circuit can be determined.
Recalling and substituting the terms in the phase angle equation, the phase angle between the current and voltage can be determined.
In an RLC series circuit, impedance is a ratio of voltage and current amplitude; by rearranging the equation, the current amplitude can be determined.
The voltage amplitude across each element can be determined as the product of the element's current and resistance or reactance.
By substituting the value in the equations, the voltage amplitude across each element can be determined.
32.8:
RLC Series Circuit: Problem-Solving
Consider an AC generator with a frequency of 50 hertz and a voltage of 120 volts. The AC generator is connected to an RLC series circuit with a 20-ohms resistor, a 0.2-henry inductor, and a 0.05-farad capacitor. Determine the impedance, current amplitude, and phase difference between the generator's current and emf.
To solve the problem, first, determine the known and unknown quantities in the problem. Recalling the reactance equation for the inductor and capacitor and substituting the values, the inductive and capacitive reactance can be determined as follows:
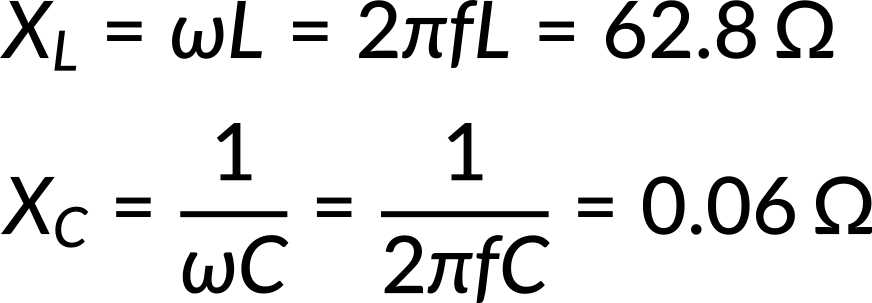
Impedance measures the combined effect of resistance, capacitive, and inductive reactance. Next, recall the impedance equation and substitute the resistance, inductive reactance, and capacitive reactance. By simplifying the equation, the impedance in the circuit can be determined as follows:
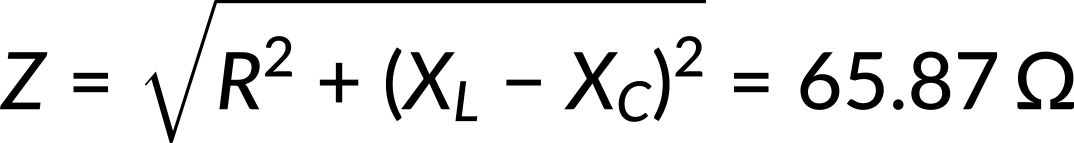
The current's amplitude is the ratio of the voltage's amplitude to the circuit's impedance. Thus, by substituting the known values, the current amplitude can be calculated as follows:

Lastly, recall the phase angle equation in the RLC circuit. By substituting the resistance and reactance values, the phase difference between the current and the emf of the generator can be calculated as follows:
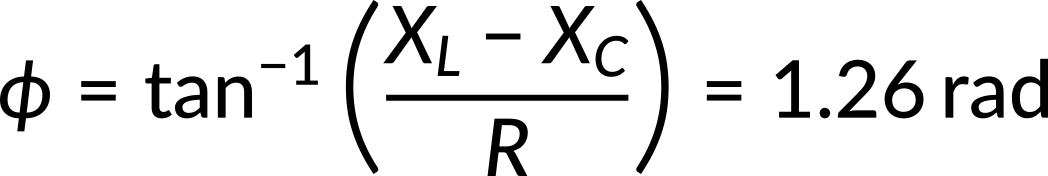
The phase angle is positive because the reactance of the inductor is larger than the reactance of the capacitor.
Suggested Reading
- OpenStax. (2019). University Physics Vol. 2. [Web version], Pg 396 – 398. Retrieved from https://openstax.org/books/university-physics-volume-2@8ede2ba/pages/15-3-rlc-series-circuits-with-ac
