14.16:
Kepler’s Second Law of Planetary Motion
Consider a planet in an elliptical orbit around the sun moving from A to A1 having an angular displacement of dθ in time dt. Then, the sector area dA covered in time dt would be half of the product of the planet's radial distance and the arc rdθ. The sector velocity dA/dt would then be equal to half of r2dθ/dt.
Here, dθ/dt is the planet's angular velocity. Thus, multiplying the equation by the planet's mass gives the sector velocity in terms of the planet's angular velocity.
However, mr2ω is the planet's angular momentum. Therefore, the sector velocity equals the ratio of the planet's angular momentum to two times its mass.
Kepler's second law states that if a planet takes the same time dt to move from B to B1, then the sector area under the arc BB1 would be equal to the area under the arc AA1.
Therefore, the sector velocity is constant, implying that the angular momentum remains conserved.
14.16:
Kepler’s Second Law of Planetary Motion
In the early 17th century, German astronomer and mathematician Johannes Kepler postulated three laws for the motion of planets in the solar system. His first law states that all planets orbit the Sun in an elliptical orbit, with the Sun at one of the ellipse's foci. Therefore, the distance of a planet from the Sun varies throughout its revolution around the Sun.
While in an elliptical orbit, the total energy of the planet is conserved. Therefore, the planet slows down when it is at apogee and speeds up when it is at perigee. These conclusions led Kepler to state his second law, that the radius vector of the planet sweeps out in equal areas in equal time. This means that if a planet takes the same time to travel from A to A1, and then from B to B1, then the areas AF1A1 and BF1B1 are equal, as shown in Figure 1.
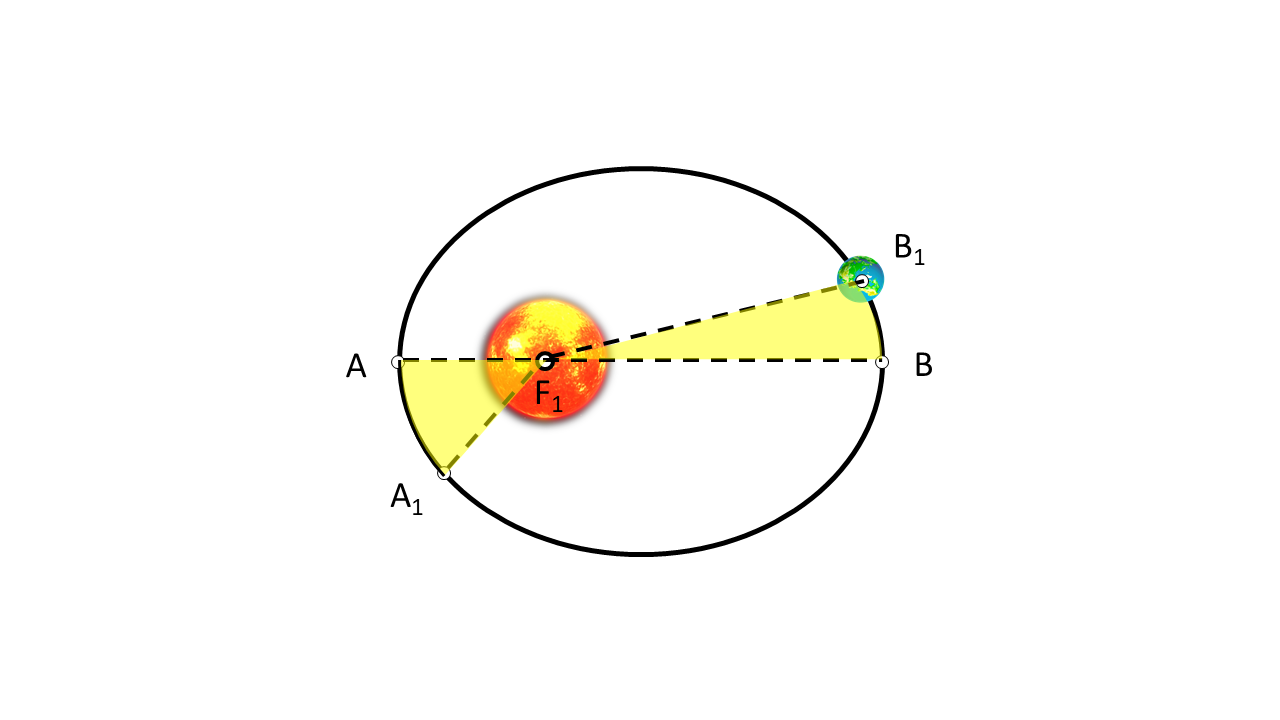
Since the area is constant in a given time interval, the planet's sector velocity remains constant. Since the sector velocity is proportional to the planet's angular momentum, Kepler’s second law implies that the angular momentum of a planet in an elliptical orbit is conserved.
This text is adapted from Openstax, University Physics Volume 1, Section 13.5 Kepler’s Laws of Motion.
