L'equazione di Nernst

Condizioni di reazione non standard

L’interconnessione tra i potenziali cellulari standard e vari parametri termodinamici come il cambiamento di energia libera standard ΔG° e la costante di equilibrio K è stata precedentemente esplorata. Ad esempio, una reazione redox che coinvolge ioni di zinco(II) e stagno(II) a 1 M di concentrazione con cella Eº = +0,291 V e ΔG° = −56,2 kJ è spontanea.

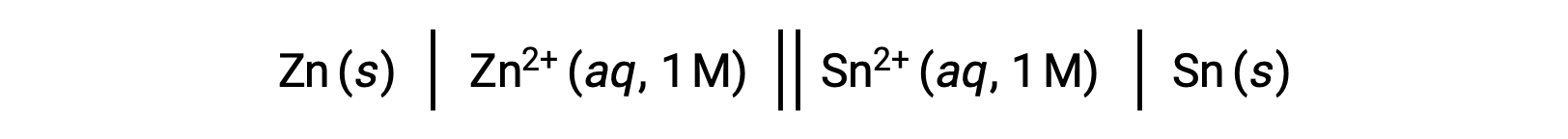
Lo scarico di questa cellula, tuttavia, comporta un cambiamento nella concentrazione del reagente e una costante diminuzione del potenziale cellulare. A tale condizione, tuttavia, le relazioni tra il potenziale cellulare e i parametri termodinamici non possono essere facilmente stabilite in quanto sono vere solo a condizioni standardizzate di concentrazione, temperatura e pressione (cioè concentrazione di 1 M, 298 K o 25 °C e una pressione di 1 atmosfera). Molte reazioni redox di significativo interesse scientifico si verificano in condizioni di stato non standard, ad esempio diverse concentrazioni di reagenti in una cellula galvanica o gradienti di concentrazione che si verificano attraverso membrane biologiche. Quindi, diventa importante calcolare le potenzialità di tali sistemi.

Quando la concentrazione di ioni di zinco nella reazione è minore e la concentrazione degli ioni stagno maggiore rispetto alle condizioni standard, la spontaneità della reazione redox può essere qualitativamente prevista usando il principio di Le Chatelier. Data la maggiore concentrazione del prodotto al reagente, la reazione ha una maggiore tendenza a procedere nella direzione favorendo la generazione dei prodotti. Ciò si traduce in un valore potenziale cellulare o una cella E_superiore a quello del valore della_cella E°.

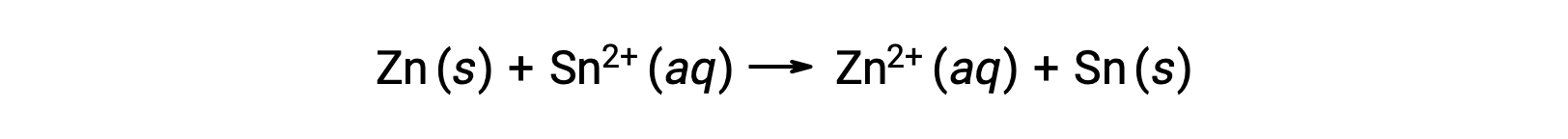
Questa reazione procede nella direzione in avanti; tuttavia, il valore quantitativo di questo potenziale cellulare non può essere facilmente determinato.

Derivazione dell’equazione di Nernst per le reazioni redox che si verificano in condizioni non standard

La relazione tra i valori_cellulari E_e E° può essere derivata dalla relazione precedentemente stabilita tra cambiamenti di energia libera a condizioni standard e non standard, che è data come segue:

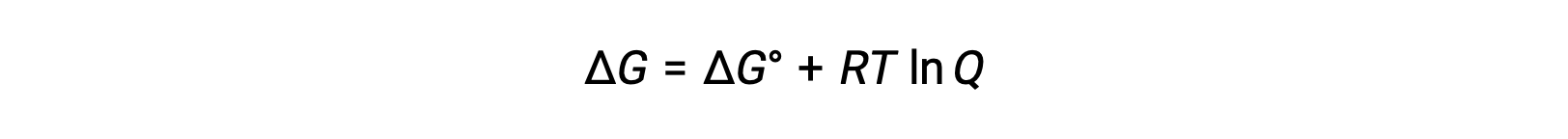
ΔG è il cambiamento nell’energia libera, ΔG° è il cambiamento standard nell’energia libera, R è la costante di gas (valore = 8,314 J/mol–K), e Q è il quoziente di reazione, che spiega la variazione di energia libera dovuta alla differenza nella composizione delle miscele di reazione. Il valore Q viene omesso se i reagenti sono solidi.

Sulla sostituzione dell’equazione relativa al cambiamento di energia libera al potenziale cellulare, si ottiene un’equazione modificata, nota come equazione di Nernst.

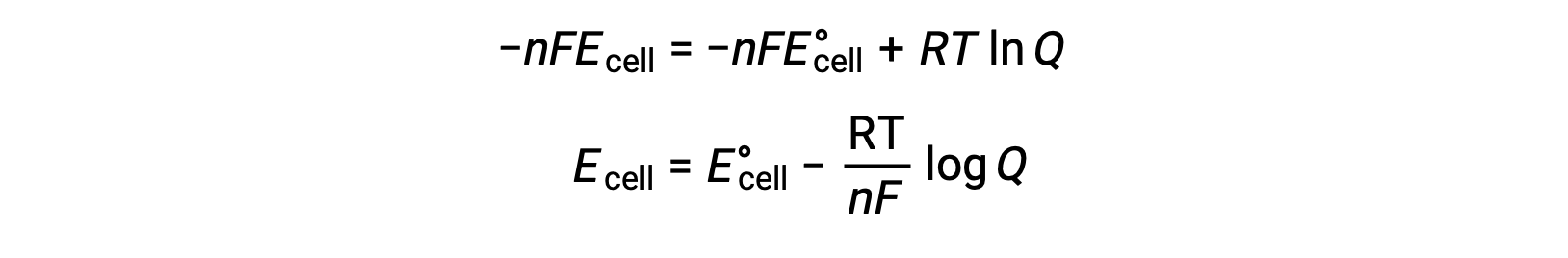
L’equazione di Nernst descrive la variazione del potenziale di un sistema redox (come una cella galvanica) dal suo valore di stato standard. Dipende dal numero di elettroni trasferiti durante la reazione redox, n , dallatemperatura misurata in kelvin, T e dalla composizione della miscela di reazione data come Q.

Una forma semplificata dell’equazione di Nernst per la maggior parte del lavoro è quella in cui sono stati inclusi i valori per le costanti fondamentali (R e F) e un fattore di conversione da log naturale a logaritmi di base-10:

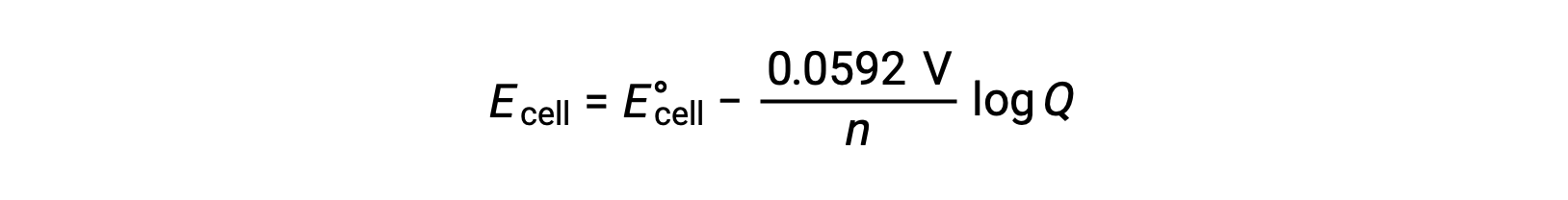
In condizioni di stato standard, il valore del quoziente di reazione Q è unità, il cui logaritmo è zero. Ciò è dovuto alla stessa concentrazione di reagenti e prodotti in condizioni di stato standard. Qui, la_cella Eè uguale alla cella E_°. Un valore Q inferiore a uno indica una maggiore concentrazione di reagenti, spostando l’equilibrio di reazione a destra e, quindi, producendo un valore più elevato del potenziale cellulare. Un valore Q maggiore di uno indica una maggiore concentrazione del prodotto, guidando la reazione a sinistra, e un valore inferiore del potenziale cellulare. All’equilibrio, il valore Q è uguale a K, e il potenziale cellulare diventa zero, cioè la reazione non mostra alcuna tendenza a procedere in entrambe le direzioni. Questo spiega perché le batterie “muoiono” a scarica continua: la diminuzione della concentrazione di reagenti spinge la reazione verso l’equilibrio e il suo potenziale cellulare diminuisce costantemente a zero.

Questo testo è adattato da Openstax, Chemistry 2e, Sezione 17.4: Potenziale, Energia Libera ed Equilibrio.